[ad_1]

The muon's magnetic moment has been a scientific mystery due to the slight difference between its theoretical and experimental values, suggesting interactions with unknown particles or forces. Research involving advanced quantum simulations is beginning to unravel these discrepancies, providing insight into the fundamental properties of muons and their interactions in particle physics. Credit: SciTechDaily.com
Researchers have identified the origin of discrepancies in recent predictions of the muon's magnetic moment. Their findings could contribute to the study of dark matter and other aspects of new physics.
A magnetic moment is an intrinsic property of a spinning particle, arising from the interaction between the particle and a magnet or other object with a magnetic field. Like mass and electric charge, magnetic moment is one of the fundamental quantities in physics. There is a difference between the theoretical value of the magnetic moment of the muon, a particle belonging to the same class as the electron, and the values obtained in high-energy experiments conducted in particle accelerators.
The difference only appears to the eighth decimal place, but scientists have been interested in it since its discovery in 1948. It's not a detail: it could indicate whether the muon is interacting with dark matter particles or other Higgs bosons, or even whether it's unknown. Troops are participating in this operation.
Inconsistencies in the muon's magnetic moment
The theoretical value of the muon's magnetic moment, represented by the letter g, is given by the Dirac equation – formulated by the English physicist and 1933 Nobel Prize winner Paulo Dirac (1902-1984), one of the founders of quantum mechanics and quantum electrodynamics – as 2. However, Experiments have shown that g is not exactly 2, and there is a great deal of interest in understanding “g-2,” that is, the difference between the experimental value and the value predicted by the Dirac equation. The best experimental value currently available, obtained with an astonishing degree of precision at the Fermi National Accelerator Laboratory (Fermilab) in the US and announced in August 2023, is 2.00116592059, with an uncertainty range of plus or minus 0.00000000022.
“Precise determination of the muon magnetic moment has become a major issue in particle physics because investigating this gap between experimental data and theoretical prediction can provide information that can lead to the discovery of some amazing new effects,” said physicist Diogo Boito, a professor at the University of São Carlos Institute of Physics. São Paulo (IFSC-USP) to FAPESP.
An article on this topic by Boito and his collaborators was published in the magazine Physical review letters.
New insights from research
“Our results have been presented at two important international events. First by me during a workshop in Madrid, Spain, and then by my colleague Martin Goltermann from San Francisco State University at a meeting in Bern, Switzerland,” said Boito.
These results identify and indicate the origin of the discrepancy between the two methods used to make current predictions for muon g-2. “There are currently two methods for determining the fundamental component of g-2. The first is based on experimental data, and the second is on computer simulations of quantum chromodynamics, or QCD, the theory that studies strong interactions between quarks. These two methods lead to very different results, which is a big problem.” He explained that until this problem is solved, we cannot investigate the contributions of possible exotic particles such as new Higgs bosons or dark matter, for example, in g-2.
The study successfully explains this discrepancy, but to understand it we need to take a few steps back and start over with a somewhat more detailed description of the muon.
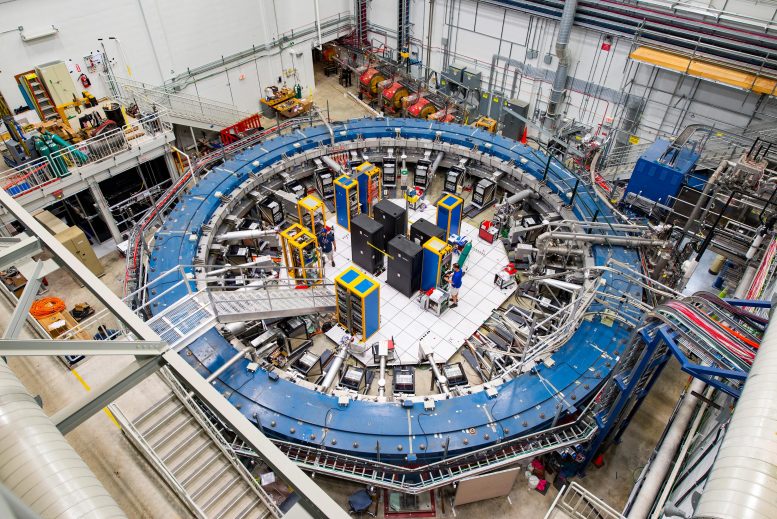
Muon storage ring at Fermilab. Credit: Reidar Hahn, Fermilab
A muon is a particle belonging to the class of leptons, as is the case with an electron, but it has a much greater mass. For this reason, it is unstable and only survives for a very short time in a high-energy context. When muons interact with each other in the presence of a magnetic field, they decay and reassemble as a cloud of other particles, such as electrons, positrons, W and Z bosons, Higgs bosons, and photons. Therefore, in experiments, muons are always accompanied by many other virtual particles. Their contributions make the actual magnetic moment measured in the experiments larger than the theoretical magnetic moment calculated by the Dirac equation, which is equal to 2.
“For the difference [g-2]it is necessary to take into account all these contributions – both those predicted by QCD [in the Standard Model of particle physics] Others are smaller in size but appear in high-precision experimental measurements. “We know many of these contributions well, but not all of them,” Boito said.
QCD strong interaction effects cannot be calculated theoretically alone, as they are impractical in some energy systems, so there are two possibilities. One has been in use for some time, and it involves resorting to experimental data obtained from electron-positron collisions, which create other particles composed of quarks. The other is lattice QCD, which has only become competitive in the current decade and entails simulating the theoretical process in a supercomputer.
“The main problem in predicting muon g-2 at the moment is that the result obtained using data from electron-positron collisions does not agree with the overall experimental result, while the results based on lattice QCD do. It was not,” Boito said. “No one is sure why, and our study explains part of this puzzle.”
He and his colleagues conducted their research specifically to solve this problem. “The article reports the results of a number of studies in which we developed a new method to compare the results of lattice QCD simulations with results based on experimental data. “We have shown that it is possible to extract with great accuracy the contributions of computed data to the lattice – contributions of so-called continuum Feynman diagrams,” he said.
American theoretical physicist Richard Feynman (1918-1988) won the Nobel Prize in Physics in 1965 (along with Julian Schwinger and Shinichiro Tomonaga) for his fundamental work in quantum electrodynamics and elementary particle physics. Feynman diagrams, created in 1948, are graphical representations of mathematical expressions that describe the interaction of these particles and are used to simplify the calculations involved.
“In this study, we obtained the contributions of continuum Feynman diagrams in the so-called 'average energy window' with great precision for the first time. Today we have eight results for these contributions, obtained by lattice QCD simulations, and they are all in great agreement. Moreover, we have shown The results based on the electron-positron interaction data do not agree with these eight results from the simulations.
This enabled researchers to identify the source of the problem and think about possible solutions. “It became clear that if the experimental data for the two-pion channel were underestimated for some reason, this could be the reason for the discrepancy,” he said. Pions are mesons, particles made up of quarks and antiquarks produced in high-energy collisions.
In fact, new data (still under peer review) from CMD-3 experience This study conducted at Novosibirsk State University in Russia seems to show that the oldest binary channel data may have been underestimated for a reason.
Reference: “Data-driven determination of the light quark-related component of the mean window contribution to the muon g−2“By Jenessa Benton, Diogo Boito, Martin Golterman, Alexander Keshavarzi, Kim Maltman, and Santiago Pires, December 21, 2023, Physical review letters.
doi: 10.1103/PhysRevLett.131.251803
Boito's participation in the study was part of his project “Testing the Standard Model: Precision QCD and muon g-2,” for which FAPESP awarded him a Phase II Young Investigator Grant.
[ad_2]
Source

